T-Line Held Strong for the SPY’s Rough Day
(SPY) The SPY had a troublesome start for most of the day, but the T-Line held strong for the SPY’s Rough Day. The Bulls still outnumber the Bears and the open looks modest, of course, I am writing this before the ADP numbers post And tomorrow we have jobs numbers.
Yesterday I mentioned the 23.6 Fibonacci number and while Fib lines are not a perfect indicator for trading yesterday’s low came within pennies of the 23.6 Fib line.
FREE TRADE IDEA – HTZ
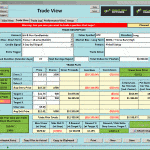 HTZ Hertz Global Holdings has been in a downtrend for some time and may be ready for a Double Digit relief rally.
HTZ Hertz Global Holdings has been in a downtrend for some time and may be ready for a Double Digit relief rally.
With on-demand recorded webinars, eBooks, and videos, member and non-member eLearning, plus the Live Trading Rooms, there is no end your education. Start small and learn
Conditions and Reasons to Trade HTZ
- Pinball Setup
- Downtrend
- Double bottom
- Hammer
- Bullish Engulf
- Over 20% possible[button_1 text=”Learn%20How%20We%20Plan%20To%20Make%20%242000%20In%20HTZ” text_size=”32″ text_color=”#000000″ text_bold=”Y” text_letter_spacing=”0″ subtext_panel=”Y” subtext=”Less%20than%20%243.00%20a%20day%20learn%20to%20trade%20independently” subtext_size=”15″ subtext_color=”#f72626″ subtext_bold=”Y” subtext_letter_spacing=”0″ text_shadow_panel=”N” styling_width=”40″ styling_height=”30″ styling_border_color=”#000000″ styling_border_size=”1″ styling_border_radius=”6″ styling_border_opacity=”100″ styling_shine=”Y” styling_gradient_start_color=”#ffff00″ styling_gradient_end_color=”#ffa035″ drop_shadow_panel=”N” inset_shadow_panel=”N” align=”center” href=”https://hitandruncandlesticks.com/hit-and-run-candlesticks/” new_window=”Y”/]
TDOC is up 40.65% from our members only post on February 28, 2017. We are about the education.
200 shares of TDOC was worth $1750.00 yesterday –
What is a Trade Idea Watch List?
A trade idea watch list is a list of stocks that we feel will move in our desired direction over a swing trader’s time frame. That time could be one to 15 days for example. From that watch-list, we wait until price action meets our conditions for a trade.
Investing and Trading involve significant financial risk and is not suitable for everyone. No communication from Hit and Run Candlesticks Inc. is not financial or trading advice. All information is intended for Educational Purposes Only. Terms of Service.
Rick Saddler is not a licensed financial adviser nor does he offer trade recommendations or advise to anyone except for the trading desk of Hit and Run Candlesticks Inc.
End of Day Rally Inspires Cautious Optimism
 The end of day rally yesterday was nice to see as the Bulls continue to defend price support. There are many that will point to the hammer candle patterns as signs of bullishness, and they could very well be correct. However, let’s not forget that the same candle pattern can also ba a Hanging Man because of its placement near all-time highs. Personally, I want to be optimistic that the Bulls are ready to push higher, but I must continue to prepare for the potential reversals. Remember price swings can happen very quickly at or near potential tops. Intraday whipsaw price action is common as highs are tested so it’s wise to be ready for additional volatility when planning new positions.
The end of day rally yesterday was nice to see as the Bulls continue to defend price support. There are many that will point to the hammer candle patterns as signs of bullishness, and they could very well be correct. However, let’s not forget that the same candle pattern can also ba a Hanging Man because of its placement near all-time highs. Personally, I want to be optimistic that the Bulls are ready to push higher, but I must continue to prepare for the potential reversals. Remember price swings can happen very quickly at or near potential tops. Intraday whipsaw price action is common as highs are tested so it’s wise to be ready for additional volatility when planning new positions.
On the Calendar
Today is a busy Economic Calendar with reports that do have the ability of move the market. It starts off with the ADP Employment Report at 8:15 Am Eastern. The ADP number last month missed the mark calling for more jobs growth than actually showed up. Today they the number is expected to be between 170k to 177K jobs created. At 8:30 AM we receive the weekly jobless claims which have been tracking at 40-year lows. The PMI Manufacturing Index comes out at 9:45 AM. PMI is expected to come in with a very strong reading of 53.0 this month.
One of the heavy hitters this morning is the ISM MFG Index number at 10:00 AM. Consensus suggests the ISM will come in steady and strong with a reading around 54.6. Construction Spending numbers also at 10:00 AM have been tracking flat this year, but today forecasters are expecting an improvement. Last but not least is the very important Oil Status Report. If we want the market to continue marching higher, we will need some help from declining surplus supplies. There are 45 companies are expected to report earnings today.
Action Plan
The end of day rally yesterday was inspiring but fell just short of providing bullish confidence. I will approach the market today with some cautious optimism. Futures are currently flat, but with all the morning economic reports quick price swings will be possible. We must also keep in mind that the market may decide to simply wait for the Employment Situation number.
[button_2 color=”green” align=”center” href=”https://youtu.be/ctMF4z-Gdco”]Morning Market Prep Video[/button_2]
Trade Wisely,
Doug
Price action indicates fear of reversal is extremely low.
 Although price action yesterday lacked energy it continues to suggest there is no fear. QQQ’s continued to dominate holding up all the other indexes and marking out yet another record high. VIX the so-called fear index is once again testing prices near historic lows. Overall market trends remain bullish, so I will continue to seek long positions but want to guard against complacency. Always be mindful around market highs that reversals are possible and can develop swiftly. Maintain focus on price action and be prepared to act no matter the direction avoiding personal bias.
Although price action yesterday lacked energy it continues to suggest there is no fear. QQQ’s continued to dominate holding up all the other indexes and marking out yet another record high. VIX the so-called fear index is once again testing prices near historic lows. Overall market trends remain bullish, so I will continue to seek long positions but want to guard against complacency. Always be mindful around market highs that reversals are possible and can develop swiftly. Maintain focus on price action and be prepared to act no matter the direction avoiding personal bias.
On the Calendar
The hump day Economic Calendar starts will the Chicago PMI at 9:45 AM Eastern followed shortly after by Pending Home Sales at 10:00 AM. The Chicago PMI has been tracking strongly above the mid-50’s for several months in a row. Although consensus is expecting a slight pullback this month, the should remain over 57 suggesting economic strength. The Pending Home Sales Index fell last month, but forecasters are expecting a rebound this month.
This afternoon at 2:00 PM Eastern we get another reading of the Federal Beige Book. We will also hear from two Fed speakers today. One at 8:00 AM and the other well after the market close at 7:30 PM. On the Earnings Calendar, there are 40 companies expected to report today. Although there is no market-moving earnings report, it is still important to continue checking before entering new trades and managing existing positions.
Action Plan
Yesterday’s light price action was as expected, but there was notable strength in several tech sector stocks. QQQ, once again claimed new record highs while the other indexes lagged behind on anemic volume. Futures has fluctuated this morning between negative and positive readings. I am hoping to see slightly more energy today but will continue to be very picky until I see buyers actively stepping in at these levels. I will continue to remain bullish if the uptrend continues to hold.
Keep in mind that with all the data points building toward the big Employment Situation report on Friday, that light volume choppiness is possible. Although I want to trade with the direction of the market, I want to be mindful not get overly long until we see price action proof that new support levels have buyers defending them.
[button_2 color=”orange” align=”center” href=”https://youtu.be/2ZvSjuzZrjM”]Morning Market Prep Video[/button_2]
Trade Wisely,
Doug
A Day OF Rest For The SPY
(SPY) – Yesterday’s price action ended up being a day of rest for the SPY giving back only .21 cents. Closing yesterday above the T-Line and the 23.6% Fibonacci retracement is a clue the Bulls are still in charge.
The leading sectors are Technology, Utilities, Consumer Defense, and Healthcare. Healthcare is currently showing signs of a possible top. Price is below recent tops and the T-Line, weakness today will drop price below the Lower T-Line Band as well.
FREE TRADE IDEA – FSLR
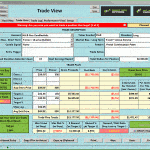 FSLR recently broke out of the downtrend line and the 200-sma. Price has made higher highs and higher lows with a Bullish J-Hook pattern off the T-Line. Start your membership
FSLR recently broke out of the downtrend line and the 200-sma. Price has made higher highs and higher lows with a Bullish J-Hook pattern off the T-Line. Start your membership
MNKD is up 20.44% from our members only post on ApMay 23, 2017. Price action and Candlesticks rule!
2500 shares of MNKD was worth $700.00 yesterday –
With on-demand recorded webinars, eBooks, and videos, member and non-member eLearning, plus the Live Trading Rooms, there is no end your education. Start small and learn
What is a Trade Idea Watch List?
A trade idea watch list is a list of stocks that we feel will move in our desired direction over a swing trader’s time frame. That time could be one to 15 days for example. From that watch-list, we wait until price action meets our conditions for a trade.
Investing and Trading involve significant financial risk and is not suitable for everyone. No communication from Hit and Run Candlesticks Inc. is not financial or trading advice. All information is intended for Educational Purposes Only. Terms of Service.
Rick Saddler is not a licensed financial adviser nor does he offer trade recommendations or advise to anyone except for the trading desk of Hit and Run Candlesticks Inc.
Sell in May and go away? Not so much!
 This year those that followed the old axiom, “Sell in May and go away”; missed out on big time. Fear of a sell remains at near record lows, and it seems even the smallest selloffs attract dip, buyers. Nothing about this year has so far has qualified as normal. As a result, we must remain very focused on price action clues and prepared for anything. That means don’t become complacent thinking that the market it too strong to sell off because that is a mistake that can remove all your profits for the year very quickly. If buyers continue to show strength at these levels, we must also be ready to act.
This year those that followed the old axiom, “Sell in May and go away”; missed out on big time. Fear of a sell remains at near record lows, and it seems even the smallest selloffs attract dip, buyers. Nothing about this year has so far has qualified as normal. As a result, we must remain very focused on price action clues and prepared for anything. That means don’t become complacent thinking that the market it too strong to sell off because that is a mistake that can remove all your profits for the year very quickly. If buyers continue to show strength at these levels, we must also be ready to act.
With no major news events over the holiday, I would expect a light volume day as many traders will have planned extended vacations.
On the Calendar
Although this is a short week, the Economic Calendar is full of very big reports. It kicks off the today with Personal Income and Outlays at 8:30 AM Eastern. Consumer is spending is expected to see an improvement over last month, but consensus suggests personal income will remain soft. At 9:00 AM we get the S&P Case-Shiller numbers followed by the Consumer Confidence reading at 10 AM.
Housing demand has been extremely strong this year bu the Case-Shiller number is expected to soften from 6% to 5.8%. Consumer Confidence is also expected to retreat ever so slightly today but continues to remain very strong and near 20-year highs. There are only 33 companies on the Earnings Calendar today, and there are none that would likely move the market.
Action Plan
Although it is likely to just a little more price action today, then we saw last Friday I am expecting much. There were not any major new events over the holiday, and the evil doers of the world behaved. Futures are pointing to a slightly soft open, but that should not be a surprise after last week’s breakout. My plan is of course to manage my current positions, but I will need to see some good price action before concerning myself with new trades. Ultimately I want to see the overall market test and hold the breakout of last week before getting too excited about adding new risk. Historically June is a month that is flat to just slightly positive. However, there is nothing about this year that has been normal, so we need to prepare for that fact that anything is possible.
[button_2 color=”green” align=”center” href=”https://youtu.be/d50XOozVaNk”]Morning Market Prep Video[/button_2]
Trade Wisely,
Doug
In the Past 7 Trading Days 7 Higher Lows
(SPY) – A Bullish T-Line Run and in the past 7 trading days 7 higher lows. Despite the Bearish resistance the Bull has managed to break through. The Harami candle printed on Friday may be suggesting the SPY needs a little rest and test.
Hit and Run Candlesticks overall plan are to remain bullish until we see a Candle Signal or a chart pattern breakdown. We often look at the 2 and three-day charts to peel off a little noise. On the three-day chart, we see a Bullish Engulf, double bottom, Bullish Morning Star, and a Bullish Harami. W hope that helps.
FREE TRADE IDEA – IMMU
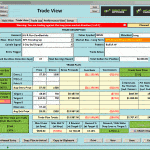 IMMU gapped out of a Bullish “W” pattern, then broke out of the March highs on a Bullish Engulf. Consolidated as it ran the T-Line. Start your membership
IMMU gapped out of a Bullish “W” pattern, then broke out of the March highs on a Bullish Engulf. Consolidated as it ran the T-Line. Start your membership
VEEV is up 27.28% from out members only post on April 21, 2017. What a great T-Line Run!
100 shares of VEEV was worth $1432 Friday – Learn the T-Line from the creator of the T-Line.
With on-demand recorded webinars, eBooks, and videos, member and non-member eLearning, plus the Live Trading Rooms, there is no end your education. Start small and learn
[button_1 text=”30-Day%20Trial” text_size=”32″ text_color=”#000000″ text_bold=”Y” text_letter_spacing=”0″ subtext_panel=”Y” subtext=”Click%20Here” subtext_size=”15″ subtext_color=”#f93939″ subtext_bold=”Y” subtext_letter_spacing=”0″ text_shadow_panel=”N” styling_width=”40″ styling_height=”30″ styling_border_color=”#000000″ styling_border_size=”1″ styling_border_radius=”6″ styling_border_opacity=”100″ styling_shine=”Y” styling_gradient_start_color=”#ffff00″ styling_gradient_end_color=”#ffa035″ drop_shadow_panel=”N” inset_shadow_panel=”N” align=”center” href=”https://hitandruncandlesticks.com/hrc-rwo-30-day-offer/” new_window=”Y”/]
What is a Trade Idea Watch List?
A trade idea watch list is a list of stocks that we feel will move in our desired direction over a swing trader’s time frame. That time could be one to 15 days for example. From that watch-list, we wait until price action meets our conditions for a trade.
Investing and Trading involve significant financial risk and is not suitable for everyone. No communication from Hit and Run Candlesticks Inc. is not financial or trading advice. All information is intended for Educational Purposes Only. Terms of Service.
Rick Saddler is not a licensed financial adviser nor does he offer trade recommendations or advise to anyone except for the trading desk of Hit and Run Candlesticks Inc.
 HTZ Hertz Global Holdings has been in a downtrend for some time and may be ready for a Double Digit relief rally.
HTZ Hertz Global Holdings has been in a downtrend for some time and may be ready for a Double Digit relief rally.







